Database of Singular Value Decompositions of Matrix Representations of the Costas Condition
- Citation Author(s):
- Submitted by:
- James Beard
- Last updated:
- DOI:
- 10.21227/h498-px29
- Data Format:
- Links:
 458 views
458 views
- Categories:
- Keywords:
Abstract
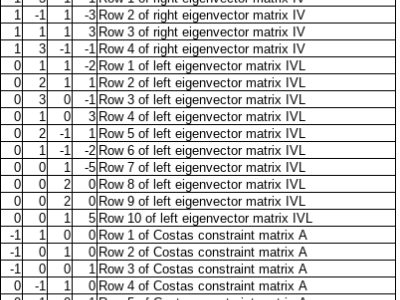
The Costas condition on a permutation matrix, expressed as row indices as elements of a vector c, can be expressed as A*c=b, where b is a vector of integers in which no element is zero. A particular formulation of the matrix A allows a singular value decomposition in which the eigenvalues are squared integers and the eigenvalues may be scaled to vectors with all integer elements. This is a database of the Costas constraint matrices A, the scaled eigenvectors, and the squared eigenvalues for orders 3 through 100.
Instructions:
Please refer to the file CC_SVD_Database_Readme.pdf for instructions on the format of the database, and its use. The database contains one file for each order. The files are CSV files in which each line ends with a comma, then a plain text remark that explains that line.
Dataset Files
- Costas condition matrix SVDs for orders 3 through 53 (Size: 5.83 MB)
- Costas condition matrix SVDs for orders 54 through 75 (Size: 20.23 MB)
- Costas condition matrix SVDs for orders 76 through 86 (Size: 20.73 MB)
- Costas condition matrix SVDs for orders 87 through 94 (Size: 21.61 MB)
- Costas condition matrix SVDs for orders 95 through 100 (Size: 20.59 MB)
- Costas condition matrix right eigenvectors & eigenvalues (Size: 17.83 MB)